Proof. The proof is technical—the idea is to write an inverse map sending each singular simplex to a smooth singular simplex, such that the assignment respects the boundary map. Then, one needs to write a homotopy from the newly constructed smooth singular simplex to the original singular simplex. ◻

Proof. First, \(D\) is clearly a graded \(R\)-module homomorphism of degree \(p_1 + p_2\), since it is the sum of compositions of \(R\)-module homomorphisms with the degrees adding up correctly. It remains to show that \(D\) is a derivation. We compute (analogously to the standard Lie bracket computation) the following expression for \(D_1D_2(ab)\), \[\begin{align*} &= D_1(D_2(a)b + (-1)^{p_2|a|}aD_2(b))\\ &= D_1 D_2(a)b + (-1)^{p_1|a|+p_1p_2} D_2(a)D_1(b) + (-1)^{p_2|a|}D_1(a)D_2(b) + (-1)^{(p_1+p_2)|a|}aD_1D_2(b), \end{align*}\] and swapping the indices we compute the following expression for \(D_2 D_1(ab)\), \[D_2 D_1(a)b + (-1)^{p_2|a|+p_1p_2} D_1(a)D_2(b) + (-1)^{p_1|a|}D_2(a)D_1(b) + (-1)^{(p_1+p_2)|a|}aD_2D_1(b).\] Finally, we get cancellation of the mixed terms by our choice of sign \((-1)^{p_1p_2}\), so indeed \(D\) is a degree \((p_1+p_2)\) \(R\)-derivation. ◻

Proof. By definition, \[\begin{align*} D(b\omega) &= D(b)\omega + b D(\omega)\\ &= D(b)\omega + b D(\omega), \end{align*}\] so evaluating at \(p\) we get \[D(b\omega)_p = D(b)_p \omega_p + b(p) D(\omega)_p = 0\omega_p + 1 \cdot D(\omega)_p = D(\omega)_p,\] since a degree \(0\) derivation on \(\Omega(M)\) restricts to a derivation \(D: C^\infty(M) \to C^\infty(M)\) which is just a vector field, which has \(D(b)_p=0\) because \(b\) is constant in a neighborhood of \(p\). ◻

Proof. Let \(\theta_t\) be the flow corresponding to \(X\). Then, \[\mathcal{L}_X(d\omega) = \frac{\mathrm d }{\mathrm d t}|_{t=0} (\theta_t)^* d\omega,\] and recalling that the exterior derivative commutes with pullbacks, we get \[\mathcal{L}_X(d\omega) = \frac{\mathrm d }{\mathrm d t}|_{t=0} d (\theta_t)^* \omega = d(\frac{\mathrm d }{\mathrm d t}|_{t=0} (\theta_t)^* \omega),\] (where the exterior derivative commutes with the time derivative by commuting mixed partial derivatives), giving us \[\mathcal{L}_X(d\omega) = d(\mathcal{L}_X \omega).\] ◻

Proof. We can recognize the right hand side as \[d \iota_X - (-1)^{\deg(d)\deg(\iota_X)} \iota_X d,\] so it is a graded commutator. Thus, by our lemma, this is a degree 0 derivation (just like \(\mathcal{L}_X\)).

To show that these two derivations agree, we first reduce to a local version of the problem. Given \(\omega \in \Omega(M)\), we need to show that for all \(p\in M\), \[(\mathcal{L}_X \omega)_p = (d\iota_X + \iota_X d)(\omega)_p.\] Choose a chart \(U\) containing \(p\), and choose a bump function \(b\) supported in \(U\) with \(b(p)=1\). Then, for any derivation \(D\), \[D(b\omega)_p = D(\omega)_p.\] Thus, we can assume that \(\omega=b\omega\) is supported in a chart. Let \(x_1,\dots,x_n\) be coordinates for \(U\), so that we can write \[\omega = \sum_I f_I dx_I,\] where \(I\) is an ordered tuple, \(dx_I\) denotes the wedge over \(I\), and each \(f_I \in C^\infty(M)\). In this way, \(\omega\) is in the subalgebra of \(\Omega(M)\) generated by \(0\)-forms and exact \(1\)-forms.

We show \(\mathcal{L}_X\) and \(d\iota_X + \iota_X d\) agree on \(0\)-forms and exact \(1\)-forms, so that they will agree on such \(\omega\) because they are both degree \(0\) derivations.

First, let \(f\in C^\infty(M)\). Then, \[\mathcal{L}_X f = X\cdot f\] and \[(d\iota_X + \iota_X d)(f) = 0 + \iota_Xdf = df(X) = X\cdot f,\] so indeed we get equality.

Next, let \(df \in \Omega^1(M)\) be an exact 1-form. Then, \[\mathcal{L}_X (df) = d(X\cdot f)\] by the lemma, and \[(d\iota_X + \iota_X d)(df) = d(df(X)) + \iota_X(0) = d(X\cdot f),\] so we get equality for exact 1-forms as well. ◻

Proof. We consider \(M\times I \subseteq M\times \mathbb{R}\) as a submanifold. Define a vector field \(S\) on \(M\times \mathbb{R}\) by \[S_{(q,s)} = (0, \frac{\partial }{\partial s}\mid_s)\] (identifying \(T_{(q,s)}M \cong T_q M \times T_s \mathbb{R}\)), so that the corresponding flow composed with the inclusion \(i_0\) gives the homotopy. We have the following picture.

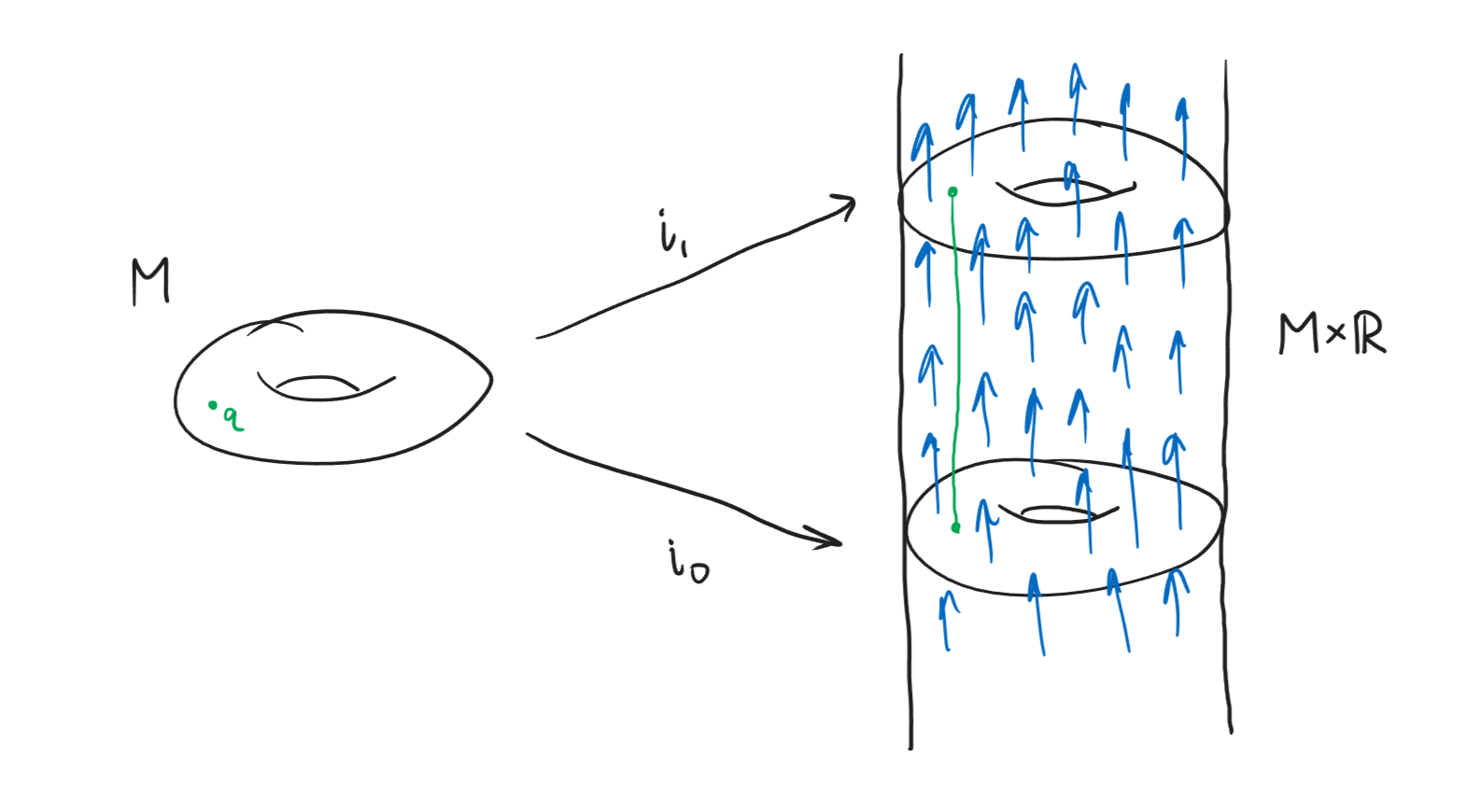
We have a vector field, now we can apply Lie’s Magic Formula. Denoting \(\theta_t\) the flow corresponding to \(S\), and replacing the Lie derivative with its flow definition, Cartan’s formula gives us \[\begin{align*} \frac{\mathrm d }{\mathrm d t}\Big|_{t=0} (\theta_t)^* &= d\iota_S + \iota_S d. \end{align*}\] Precomposing both sides with \(i_t^*\) and using the fact \(i_t = \theta_t \circ i_0\) (from the definition of our vector field), we get \[\begin{align*} (i_t)^* \frac{\mathrm d }{\mathrm d t}\Big|_{t=0} \theta_t^* &= i_t^* d\iota_S + i_t^* \iota_S d\\ \frac{\mathrm d }{\mathrm d t}\Big|_{t=t} i_0^* \theta_t^* &= d i_t^* \iota_S + i_t^* \iota_S d\\ \frac{\mathrm d }{\mathrm d t}\Big|_{t=t} i_t^* &= d i_t^* \iota_S + i_t^* \iota_S d \end{align*}\] and then integrating with respect to \(t\), we have, by the fundamental theorem of calculus \[\begin{align*} i_1^* - i_0^* &= \int_0^1 (d i_t^* \iota_S + i_t^* \iota_S d) dt. \end{align*}\] Now, we can commute the integral with the exterior derivative by differentiating under the integral, to get \[i_1^* - i_0^* = d \int_0^1 (i_t^* \iota_S) dt + \int_0^1 (i_t^* \iota_S d) dt,\] so we should define our homotopy operator \(h:\Omega^p(M\times I) \to \Omega^{p-1}(M)\) by sending \(\omega\in \Omega^p(M\times I)\) \[\omega \longmapsto \int_0^1 (i_t^* \iota_S \omega) dt.\] ◻

Proof. Let \(H:M\times I \to N\) be the (not necessarily smooth) homotopy from \(F\) to \(G\). By a corollary of the Whitney approximation theorem, we can assume \(H\) is smooth. Now, we can write the composition

By the previous theorem, \(i_0^* = i_1^*\), so we get \[\begin{align*} i_0^* \circ H^* &= i_1^* \circ H^*\\ (H \circ i_0)^* &= (H \circ i_1)^*\\ F^* &= G^*. \end{align*}\] ◻

Proof. Standard algebraic topology proof. ◻

Proof. By definition, \(H^0(\{q\}) = \ker(d: C^\infty(\{q\})\to \Omega^1(\{q\})) / \mathop{\mathrm{im}}0\), which is \(\mathbb{R}\) since the functions on \(\{q\}\) are constant functions \(\mathbb{R}\) which have differential 0. Then, \(H^p(\{q\})=0\) for \(p\geq 1\) since the cohomology groups are quotients of subobjects of \(\Omega^p(\{q\})\), which is 0 already. ◻

Proof. Homotopy invariance + de Rham cohomology of a point. ◻

Proof of (a).. This is routine. Let \([\omega]\in H_\mathrm{dR}^p(N)\). To show \(F^*\circ I([\omega]) = I\circ F^*([\omega])\), we show both evaluate the same on smooth simplices. Let \(\sigma\in C_p^\infty(N)\). Then, \[\begin{align*} (I\circ F^*)([\omega])(\sigma) &= I([F^*\omega])(\sigma)\\ &= \int_\sigma F^* \omega\\ &= \int_{\Delta^p} \sigma^*F^*\omega\\ &= \int_{\Delta^p} (F\circ \sigma)^*\omega\\ &= \int_{F\circ \omega} \omega\\ &= (F^*\circ I)([\omega])(\sigma). \end{align*}\] ◻

Proof of (b).. The key is to remember how to compute \(\delta\) and \(\partial^*\), which requires us to remember how the Zig-Zag lemma works and to go back to the SES sequences of chain complexes in de Rham/singular (co)homology which gave us Mayer-Vietoris.

As before, let \([\omega] \in H_\mathrm{dR}^{p-1}(U\cap V)\), and let \([\sigma]\in H_p^\infty(M)\). We will show \[(\partial^* \circ I)([\omega])(\sigma) = (I\circ \delta)([\omega])(\sigma).\] We first compute \((\partial^* \circ I)([\omega])(\sigma)\), using the fact that \(\partial^*\) is really dual to \(\partial_*\) (the connecting homomorphism in homology Mayer-Vietoris), so \[(\partial^* \circ I)([\omega])(\sigma) = I([\omega])(\partial_*\circ \sigma).\] Now, we recall how to compute \(\partial_*\) (going through the Zig-Zag Lemma):

• We write \(\sigma = \tau + \tau'\) for some \(\tau\in C_p^\infty(U)\) and \(\tau'\in C_p^\infty(V)\).

• We apply boundary to get the pair \((\partial\tau, \partial\tau') \in C_{p-1}^\infty(U)\oplus C_{p-1}^\infty(V)\).

• We recognize that \((\partial\tau, \partial\tau')\) come from a chain \(\tilde{\sigma} \in C_{p-1}^\infty(U\cap V)\), i.e., \([\partial\tau]=[\partial\tau']=[\tilde{\sigma}]\)

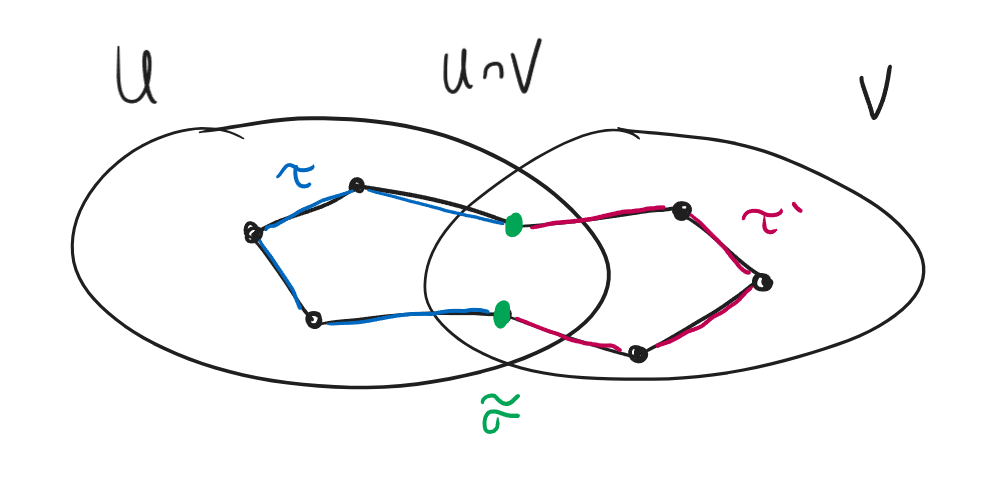
Therefore, we have \[\begin{align*} (\partial^* \circ I)([\omega])(\sigma) &= I([\omega])(\tilde{\sigma})\\ &= \int_{\tilde{\sigma}} \omega \end{align*}\]

Now, we compute \((I\circ \delta)([\omega])(\sigma)\). By definition of \(\delta\), we do the following.

• We choose a preimage of \(\omega\) under \(i_1^* - i_2^*\), say \(\omega = \eta_{U\cap V} - \eta'_{U\cap V}\) for \(\eta\in \Omega^{p-1}(U)\) and \(\eta'\in \Omega^{p-1}(V)\).

• Then, we apply the exterior derivative (the coboundary map) to get \(d\eta + d\eta' \in \Omega^p(U)\oplus \Omega^p(V)\).

• Finally, it just turns out (i.e., the Zig-Zag lemma proves) that \(d\eta,d\eta'\) comes from a differential form \(\tilde{\omega}\in \Omega^P(M)\).

Then, \(\delta([\omega]) = [\tilde{\eta}]\). Therefore, we have \[\begin{align*} (I\circ \delta)([\omega])(\sigma) &= I([\tilde{\eta}])(\sigma)\\ &=\int_\sigma \tilde{\omega}\\ &=\int_\tau \tilde{\omega} + \int_{\tau'} \tilde{\omega} \tag{$\sigma=\tau+\tau'$}\\ &= \int_\tau d\eta + \int_{\tau'} d\eta' \tag{$\tilde{\omega}$ restricted}\\ &= \int_{\tilde{\sigma}} \eta + \int_{\tilde{\sigma}} \eta' \tag{Stokes' theorem}\\ &= \int_{\tilde{\sigma}} (\eta + \eta')\\ &= \int_{\tilde{\sigma}} \omega \tag{$\omega=\eta+\eta'$} \end{align*}\] ◻

Proof. In the style of induction, our task is to show for all \(M\), \(I: H_\mathrm{dR}^p(M) \to H^p(M;\mathbb{R})\) is an isomorphism, starting with the “easy” ones.

Terminology. We call a manifold \(M\) de Rham if \(I\) is an isomorphism. We call an open cover \(\{U_i\}\) a de Rham cover if each intersection of \(U_i\) is de Rham. We write \(H^{p-1}(M)\) to be singular cohomology of \(M\) with real coefficients. We call a collection \(\{U_i\}\) a de Rham basis if it is a basis and a de Rham cover.

Strategy. Our base case is convex open subsets. Then, we will show to

Step 1. (Base Case, every convex open subset of \(\mathbb{R}^n\) is de Rham) By the Poincaré lemma, \(H_\mathrm{dR}^p(U)\) is trivial when \(p\neq 0\), which agrees with singular cohomology. We only have to show that \[I: H_\mathrm{dR}^0(U) \to H^0(U;\mathbb{R})\] is an isomorphism, i.e., not the zero map. Let \(f\in \Omega^0(U)\) be a closed form representing a generator \(H_\mathrm{dR}^0(U)\), i.e., a function which is constant (closed on a contractible set) and nonzero. Then, the 0th smooth singular homology of \(U\) is represented by any point \(x\) of \(U\), and \[I([f])([x]) = \int_{x} f = f(x) \neq 0,\] so \(I([f])\neq 0\), so \(I\) is an isomorphism.

Step 2. (Finite Induction, if \(M\) has finite de Rham cover, then \(M\) is de Rham) Suppose \(M=U\cup V\) for \(U,V,U\cap V\) de Rham manifolds. We write down the respective Mayer-Vietoris sequences for both de Rham/singular cohomology, and we write the \(I\) map going down. By the previous section, all of these squares commute, so we have the following commutative diagram with exact rows:

Now, because \(U,V\) are de Rham manifolds, this means every map downwards is an isomorphism, except for potentially the middle map. However, then by the Five Lemma, the middle map is an isomorphism, so \(M\) is a de Rham manifold.

We can use this step repeatedly to show that any \(M\) with a finite de Rham cover is a de Rham manifold.

Step 3. (Infinite-ish Induction, if \(M\) has a de Rham basis, then \(M\) is de Rham) Let \(\{U_i\}\) be a de Rham basis, and let \(f:M\to \mathbb{R}\) be an (any) exhaustion function. For each \(m\in \mathbb{N}\), define \[\begin{align*} A_m &= f^{-1}([m,m+1])\\ A_m' &= f^{-1}((m-\frac{1}{2}, m+\frac{3}{2})). \end{align*}\] so that \(M=\bigcup_{m\in \mathbb{N}} A_m\) is this union of compact sets, and each \(A_m'\) is an open neighborhood of the compact \(A_m\).

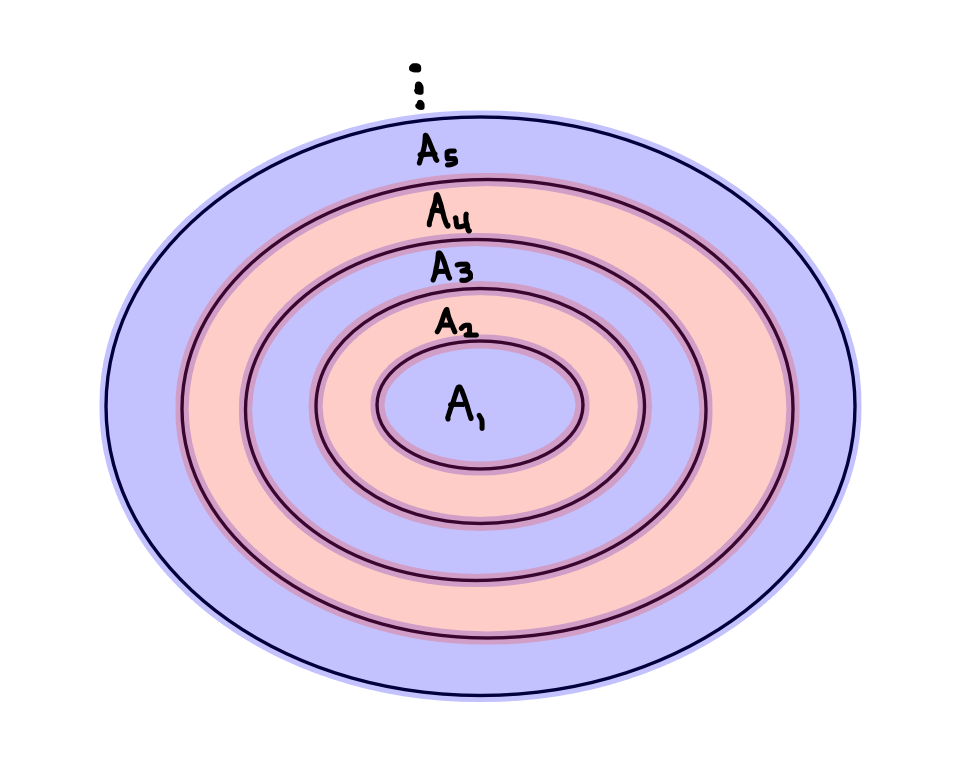
Since \(M\) has a de Rham basis, each point in \(A_m'\) has a de Rham neighborhood, and we can take a finite subcover over \(A_m\) to get a finite de Rham cover of \(A_m\), entirely contained in \(A_m'\). By Step 2, the union of these open sets \(B_m\) is de Rham.

In total, we have these “stripes” of de Rham sets, \(B_1,B_2,B_3,\dots\), and we split the stripes by \[U = B_1 \sqcup B_3 \sqcup \cdots, \qquad V = B_2 \sqcup B_4 \sqcup \cdots,\] where the unions are disjoint because, for example, \(B_1 \subseteq A_1'\) and \(B_3\subseteq A_3'\), but \(A_1'\cap A_3'=\emptyset\).

Now, because the unions are disjoint, and both cohomologies send countable infinite unions to countable infinite direct products in a way commuting with \(I\), we have that \(U,V\) are both de Rham. Furthermore, \(U\cap V\) is de Rham because each intersecting stripe is de Rham (as in the construction of the \(B_m\)), and again it is a countable disjoint union of these stripes.

Thus, by step 2, \(M=U\cup V\) is de Rham.

Step 4. (Apply the Induction) First, any open subset (not necessarily connected!) of \(\mathbb{R}^n\) is de Rham, since it has a basis of open balls which is a de Rham basis. Next, every manifold has a basis of coordinate charts (not necessarily connected!), where each susbset is diffeomorphic to an open subset of \(\mathbb{R}^n\) so is de Rham, and every finite intersection is still a coordinate chart, so still de Rham. This is therefore a de Rham cover, so \(M\) is de Rham. ◻